Measuring uncertainty in a Cubis® II laboratory balance

The QApp Pharma Package of Sartorius’s Cubis^® II premium balances contains the “Measuring Uncertainty” QApp software function.

Image credit: Sartorius

Based on the EURAMET Calibration Guide No. 18 “Guidelines on the Calibration of Non-Automatic Weighing Instruments”^1–3 (represented as “EURAMET cg-18”), this QApp function offers a dynamic display of the measurement certainty.

When the balance is in use, the attachment to Sartorius’s calibration certificate according to EURAMET cg-18 also displays an identification of weighing uncertainty.

“Measuring uncertainty,” the global expanded software function, is represented in the form of a straight-line equation, having both a constant contribution and a proportional contribution to the reading.In most cases, this indicates a considerably good assessment of the uncertainty of weighing results of the balance that is in use.

To benefit the user, the software function enables entering the parameters (as depicted in the calibration certificate based on EURAMET cg-18) of the straight-line equation into the device directly. Therefore, the software function always mentions the uncertainty for each measured value, as revealed in the appendix to the calibration certificate.

Why is measurement of uncertainty important?

Present day’s high-efficiency laboratory balances support users in all aspects, with commendable regulatory and practical functionality. However, high-efficiency laboratory balances are complicated and highly sensitive measuring devices.

Thus, based on the device, influences such as the installation location, the expertise of the user, etc., can impact the results shown.

Although the balance is leveled, calibrated and adjusted properly, the user is still left with one unanswered question: How big is the uncertainty of the weight value shown?

A dynamic function shows the uncertainty for every quantified value in the complete weighing range, which is from zero to the maximum capacity.

Customers working in regulated environments — for whom documentation of weighing values has a vital significance — benefit from this function. The function also enables printing of every weighing value with its matching uncertainty.

Sartorius Service can effortlessly set the parameters. Considering multiple parameters accounting for the uncertainty, values are taken from the calibration certificate.

In the EURAMET cg-18, all influencing parameters are elaborately described and defined. The EURAMET (European Association of National Metrology Institutes) is a joint alliance of national metrological organizations from member states of the EU (European Union) and the EFTA (European Free Trade Association).

EURAMET organizes metrological activity at a European level, communicating with the OIML (International Organization of Legal Metrology) and the BIPM (International Bureau of Weights and Measures), as appropriate.

The software function's calculation for measuring weighing results’ uncertainty comprises uncertainty contributions in many respects, for example, repeatability, rounding at zero and under load, deviation from eccentric loading, potential changes to the balance and buoyancy effects of temperature variation at the place of use. Moreover, the indication error and its interpolation are determined during calibration.

Theoretical background

The EURAMET cg-18 outlines the background of the measuring uncertainty of weighing and complete metrological theory. Further, the researchers attempt to summarize the comprehensive theory in its fundamental statements and try to clarify the essential relationships.

A typical explanation of the potential influences on the weighing result W could be demonstrated with the following equation, taking several corrections into consideration:

W = W* + δR_instr + δR_proc

In this equation, the indicated weight value right after calibration of the balance is given as W*. When the instrument is in use after calibration, the δR_instr represents the correction owing to the environmental influence. δR_proc represents all corrections due to the instrument’s operation.

To distinguish from the indications achieved during the calibration and the results achieved while weighing load (L) after the instrument’s calibration, the parameters R₀ and R_L are considered.

R₀ = The reading obtained after calibration, without load on the calibrated instrument

R_L = The reading obtained after calibration when weighing a load (L) on the calibration instrument

The weight value indicated — achieved directly after the calibration — can be expressed with the following equation:

W* = R_L + δR_digL + δR_rep + δR_ecc - (R₀ + δR_dig0) - E

The below-mentioned error contributions of the reading are taken into consideration.

δR_digL = The rounding error at load reading

δR_ecc = The error due to the eccentric position of a load

δR_dig0 = The rounding error at zero reading

δR_rep = The repeatability of the instrument

E = The indication error of a reading, reported in the calibration certificate

Usually, the instrument's user should know that the weight is different in normal use than that at calibration, in some or most cases.

The following equation represents the errors with respect to the individual environmental impacts after the calibration of the instrument, during weighing.

δR_instr = δR_temp + δR_buoy + δR_adj

δR_temp = The potential change in the instrument’s characteristics as a result of ambient temperature change

δR_buoy = The potential change in buoyancy due to the change of air density

δR_adj = The potential change in the instrument’s characteristics from the calibration time due to wear and tear or drift, which is not considered in the calibration certificate.

The contributing terms for the errors caused by the instrument’s particular operation:

δR_proc = δR_Tare + δR_time + δR_ecc

Where,

δR_Tare = The potential change owing to the tare balancing operation

δR_time = Creep’s and hysteresis’ possible effects, which are not considered in the calibration certificate

δR_ecc = The error due to the eccentric position of a load

Lastly, the expanded global uncertainty measurement U_gl (W) can be measured using the following equation that includes all the above-explained parameters.

U_gl (W) = 2 • [u²(R) + a₁² • R² + a₁² • u²(R) + R² • u²(a₁) + u² (δR_temp) + u²(δR_buoy) + u²(δR_adj) + u²(δR_Tare) + u²(δR_time) + u²(δR_ecc)]^0.5

The equation includes an extra coefficient a₁ with its uncertainty mentioned as u (a₁), which results from a linear regression of the error of the result obtained during calibration.

Uncertainty can be approximated by a straight-line equation as all contributions are either constant or proportional to R and U_gl (W).

The contributions to the global expanded uncertainty equation can be grouped into α_gl and β_gl, including constant uncertainties and proportional uncertainties, respectively. In a simple linear equation, it can be expressed as

U_gl (W) ≈ α_gl + β_gl • R

Here, the displayed weight value is R, interception  α_gl = U_gl (W = 0) , and the slope

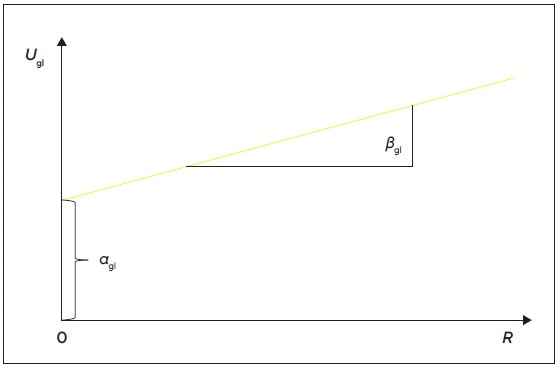
Figure 1. Graphical representation of the absolute measurement uncertainty as a straight line with interception α_gl and slope β_gl. Image Credit: Sartorius Lab Instruments GmbH & Co. KG

Download the full "Measuring Uncertainty" White Paper here to read on

References

1. EURAMET Calibration Guide No. 18, Version 4.0. Guidelines on the Calibration of Non-Automatic Weighing Instruments. (2015)
2. Sartorius White Paper: Calibration certificates according to EURAMET cg-18 (Understanding calibration certificates and practical application of the results). 2020 (planned)
3. Sartorius White Paper: Calibration guide EURAMET cg-18 for electronic non-automatic weighing instruments (Specifications, options and implementation of the guideline by Sartorius). (2020) (planned)
4. Sartorius White Paper: Minimum net weight values according to USP <41>, OIML R76 and EURAMET cg-18 (What is the minimum weight of a sample to get reliable weighing results?). (2020)

About Sartorius Lab Instruments GmbH & Co. KG

The Sartorius Group is a leading international partner of life science research and the biopharmaceutical industry.

With innovative laboratory instruments and consumables, the Group’s Lab Products & Services Division concentrates on serving the needs of laboratories performing research and quality control at pharma and biopharma companies and those of academic research institutes.

The Bioprocess Solutions Division with its broad product portfolio focusing on single-use solutions helps customers to manufacture biotech medications and vaccines safely and efficiently. The Group has been annually growing by double digits on average and has been regularly expanding its portfolio by acquisitions of complementary technologies.

In fiscal 2021, the company earned sales revenue of some 3.45 billion euros. At the end of 2021, nearly 14,000 people were employed at the Group’s approximately 60 manufacturing and sales sites, serving customers around the globe. 

Sponsored Content Policy: News-Medical.net publishes articles and related content that may be derived from sources where we have existing commercial relationships, provided such content adds value to the core editorial ethos of News-Medical.Net which is to educate and inform site visitors interested in medical research, science, medical devices and treatments.